Heptadecagons!
Today I discovered a lovely little result. If you list the vertices of a non-self-intersecting polygon in counterclockwise order, then the area of the polygon is
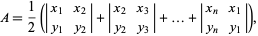
where vertical bars denote the determinant. The first thing I thought when I saw this equation was that I could use the nth roots of unity to get the vertices of regular n-gons inscribed in the unit circle, calculate their areas, and get a sequence that has pi as its limit!
Excited?
Yeah, me too!
Since this blog is called Heptadecagon, here's the inscribed heptadecagon:
At this scale, it doesn't look too much different than the circumscribed circle, but you can see all 17 distinct sides. The area of the polygon, calculated with the determinant equation, is 3.07055 to six decimal points.
Here are the results of the area sequence, from n=3 to n=1002. At 1002, the area is 3.14157. At n=20000, the area is 3.14159260, which misses pi by about five hundred millionths. If I calculate any more of these, it won't be on this machine. This old 800 MHz, Pentium III has had about all it can take.
Yes, the heptadecagon is a Matlab figure, while the area sequence curve comes from LabView. I'm bilingual.
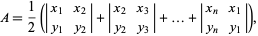
where vertical bars denote the determinant. The first thing I thought when I saw this equation was that I could use the nth roots of unity to get the vertices of regular n-gons inscribed in the unit circle, calculate their areas, and get a sequence that has pi as its limit!
Excited?
Yeah, me too!
Since this blog is called Heptadecagon, here's the inscribed heptadecagon:
At this scale, it doesn't look too much different than the circumscribed circle, but you can see all 17 distinct sides. The area of the polygon, calculated with the determinant equation, is 3.07055 to six decimal points.Here are the results of the area sequence, from n=3 to n=1002. At 1002, the area is 3.14157. At n=20000, the area is 3.14159260, which misses pi by about five hundred millionths. If I calculate any more of these, it won't be on this machine. This old 800 MHz, Pentium III has had about all it can take.
Yes, the heptadecagon is a Matlab figure, while the area sequence curve comes from LabView. I'm bilingual.
Comments
I mean, I got an a in calculus and chemistry, so historically I'm not a complete idiot, but still... just reading that is making me access parts of my brain that are crying out in pain from years of inactivity.
AND I have heard Tim make a Macbeth reference, so I can't even pretend he's one of those math and science only guys. Sheesh!
P.S. Aaron's smarter than I am already isn't he?